The Desarguesian projective plane is the only known (finite) projective plane with a group of automorphisms that acts transitively on the points of this plane. It has long been conjectured that this property characterises
:
Conjecture: A finite projective plane with a point-transitive automorphism group is Desarguesian.
But who made this conjecture? And how “long-standing” is it?
The study of exactly which conditions on the group of a projective plane characterise goes back 60 years or more. In the 1950s, Ostrom investigated projective planes with a doubly transitive automorphism group, and a famous paper of Ostrom & Wagner in 1959 completed the proof that
is the only plane with a doubly transitive automorphism group (on points). As far as I can see, while they raise some other questions about translation planes they don’t mention the conjecture at all.
A doubly transitive group of automorphisms of a projective plane is necessarily flag-transitive (that is, transitive on incident point-line pairs) but not conversely, and so the next question tackled was whether this weaker condition also characterises . In 1960, Higman and McLaughlin explicitly asked the question of whether a projective plane with a flag-transitive group must be Desarguesian (although they used the term acutely transitive) and proved a number of consequences of this including the fact that the group acts primitively on both the points and the lines of the projective plane. As far as I can see, they also did not mention anything about the question with only the hypothesis of transitivity.
The first explicit mention that I can find of the conjecture is in Dembowski’s seminal 1968 book “Finite Geometries”. He says “We begin with the case where is transitive on the points (and hence lines) of
. It has been conjectured that
must then be Desarguesian” with no attribution.
The major theorems classifying the structure of primitive permutation groups emerged from the classification of finite simple groups within the next 15 years. By exploiting the fact that flag-transitivity implies primitivity and grinding through the possible primitive groups Kantor (1985) showed that a flag-transitive projective plane is either Desarguesian or is a cyclic plane of prime order (that is, it has a cyclic group of prime order acting on it). This latter condition is very restrictive and implies various other numerical conditions that were exploited by other researchers to show that any such non-Desarguesian plane must be huge and so we’re unlikely to stumble upon one. Everyone pretty much assumes it can’t exist but we just can’t quite prove it. John’s recent post gives more details on the numerics involved. Kantor seems a little embarrassed at his approach stating “The proof of the Ostrom-Wagner theorem is both elegant and informative. By contrast, our proof … uses a sledgehammer approach” and “The tedious and somewhat ludicrous details are given in …“. But again, as far as I can see, he does not mention or allude to the transitive plane conjecture.
The most recent work on transitive planes is that of Nick Gill who, in his 2005 thesis, proved some strong results about the possible structure of a group acting transitively on a non-Desarguesian projective plane, though still not strong enough to eliminate the possibility. He is sure of how long the conjecture has been around – “For us a motivating conjecture of some fifty years standing is that all point-transitive planes are Desarguesian” – but also does not attach a name to it!
The last remaining hope is to ask some of the finite geometers / design theorists who were working in the 1950s / 1960s and see if they can remember where they heard it or to whom is was attributed. Obviously these people are somewhat thin on the ground, but Dan Hughes was gracious enough to answer my query, stating
I ‘knew’ this conjecture as early as the early ’50s, since Bruck, my supervisor, mentioned it as one of the interesting open problems. I have since heard it of it so often that, as a conjecture — or even as an open problem — it has probably earned the status of ‘folklore.
So unless anyone else can help, I guess we’ll just have to go back to the original assertion: that it is indeed a ‘”long-standing conjecture”.
One final word of warning from Dan if you are tempted to give the problem itself a go!
Good luck — but I always told my students not to work on that problem!
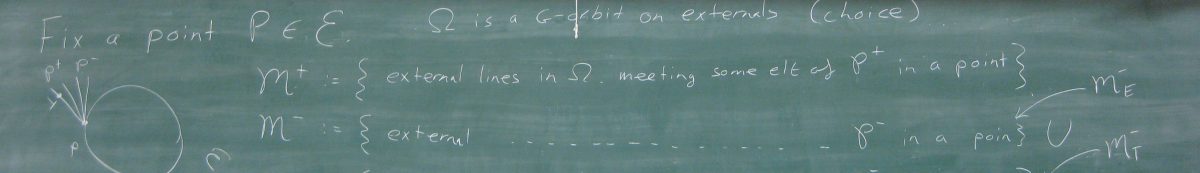
I can find an earlier reference in print. In Richard Roth’s “Flag-transitive projective planes of even order”, Proc. Amer. Math. Soc. 15(1964), 485-490, on page 486 he says “It is known that a doubly
transitive finite plane is Desarguesian…and conjectured that a transitive plane is Desarguesian.”
He gives a references for the doubly transitive theorem, but none for the conjecture.
I would suggest that the conjecture was made at a talk, rather than in print, and I would put the date
between 1959 and 1964. (Dan Hughes mentioned that Dick Bruck said only that it was an interesting open problem, not a longstanding conjecture.) People who might have conjectured it, in my opinion, include Marshall Hall, Jr., Otto Wagner and Ted Ostrom. Section 7 of Wagner’s 1959
paper “On perspectivities of finite projective planes” is entitled “Transitive projective planes”. In it,
he says “It is not known whether every transitive finite plane is desarguesian”. It seems likely
to me that he knew of no conjecture at this date.
I just learnt (at the AGT Summer school in Rogla, Slovenia) of a very interesting result of Evdokimov and Ponomorenko (2000):
The coherent configuration of a finite projective plane is schurian if and only if the projective plane is Desarguesian.
Which result of Evdokimov and Ponomarenko do you mean? There is a paper (published in 2000) in Electronic J. Comb, where they have a result about Schurianness of the coherent configuration of points and lines of the plane, but this is more or less immediate from Ostrom-Wagner Theorem.
I’ve found another, even earlier, mention:
Hughes, D. R.
Review of some results in collineation groups. 1959 Proc. Sympos. Pure Math., Vol. 1 pp. 42–55 American Mathematical Society, Providence, R.I.
On page 52, Dan Hughes says:
“Indeed if it has a transitive collineation group, then [a finite projective plane] is probably Desarguesian.”